Fixed-pinned beam - Couple moment Mo at any point Calculator
Formula
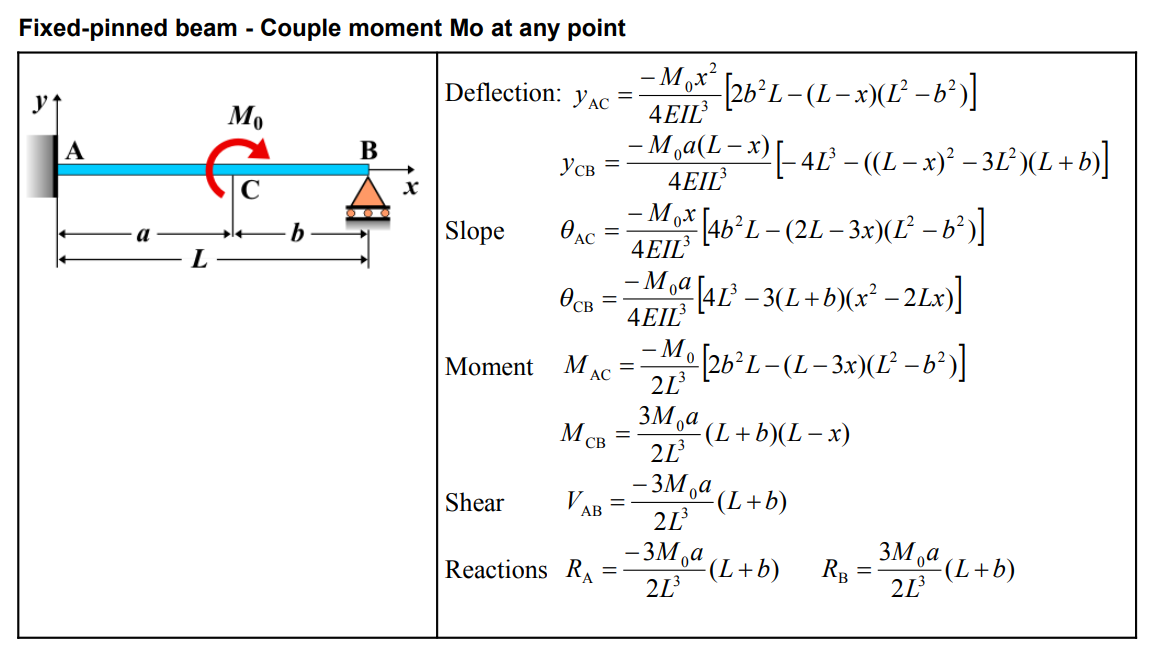
Beam Formulas
Beam Deflection, Slope, Moment, Shear, and Reactions
| Property |
Formula |
| Deflection (\(y_{AC}\)) |
\(y_{AC} = \frac{-M_0 x}{4EI L^3} \left[ 2b^2 L - (L-x)(L^2 - b^2) \right]\) |
| Deflection (\(y_{CB}\)) |
\(y_{CB} = \frac{-M_0 a(L-x)}{4EI L^3} \left[ -4L^3 - ((L-x)^2 - 3L^2)(L+b) \right]\) |
| Slope (\(\theta_{AC}\)) |
\(\theta_{AC} = \frac{-M_0 x}{4EI L^3} \left[ 4b^2 L - (2L-3x)(L^2 - b^2) \right]\) |
| Slope (\(\theta_{CB}\)) |
\(\theta_{CB} = \frac{-M_0 a}{4EL^3} \left[ 4L^3 - 3(L+b)(x^2 - 2Lx) \right]\) |
| Moment (\(M_{AC}\)) |
\(M_{AC} = \frac{-M_0}{2L^3} \left[ 2b^2 L - (L-3x)(L^2 - b^2) \right]\) |
| Moment (\(M_{CB}\)) |
\(M_{CB} = \frac{3M_0 a}{2L^3} (L+b)(L-x)\) |
| Shear (\(V_{AB}\)) |
\(V_{AB} = \frac{-3M_0 a}{2L^3} (L+b)\) |
| Reaction (\(R_A\)) |
\(R_A = \frac{-3M_0 a}{2L^3} (L+b)\) |
| Reaction (\(R_B\)) |
\(R_B = \frac{3M_0 a}{2L^3} (L+b)\) |
Definitions
| Symbol |
Physical quantity |
Units |
| E·I |
Flexural rigidity |
N·m², Pa·m⁴ |
| y |
Deflection or deformation |
m |
| θ |
Slope, Angle of rotation |
- |
| x |
Distance from support (origin) |
m |
| L |
Length of beam (without overhang) |
m |
| M |
Moment, Bending moment, Couple moment applied |
N·m |
| P |
Concentrated load, Point load, Concentrated force |
N |
| w |
Distributed load, Load per unit length |
N/m |
| R |
Reaction load, reaction force |
N |
| V |
Shear force, shear |
N |

 Home
Home Back
Back